Pattern modifiers are statements or parameters which modify how a pattern is evaluated or tells what to do with the pattern. The complete syntax is:
turbulence octaves Count | omega Amount | lambda Amount |
warp { } |
frequency phase Amount |
ramp_wave triangle_wave | sine_wave | scallop_wave |
cubic_wave poly_wave [Exponent]
agate_turb brick_size mortar Size
interpolate control0 control1 Value
color_map{ } | colour_map{ COLOR_MAP_BODY } |
pigment_map{ } |
quick_color quick_colour COLOR
normal_map{ } |
slope_map{ } |
bump_size texture_map{ }
color_map{ } | colour_map{ COLOR_MAP_BODY } |
density_map{ }
The modifiers PIGMENT_LIST, quick_color, and pigment_map apply only to pigments. See section "Pigment" for details on these pigment-specific pattern modifiers.
The modifiers COLOR_LIST and color_map apply only to pigments and densities. See sections "Pigment" and "Density" for details on these pigment-specific pattern modifiers.
The modifiers NORMAL_LIST, bump_size, slope_map and normal_map apply only to normals. See section "Normal" for details on these normal-specific pattern modifiers.
The TEXTURE_LIST and texture_map modifiers can only be used with patterned textures. See section "Texture Maps" for details.
The DENSITY_LIST and density_map modifiers only work with media{density{..}} statements. See "Density" for details.
The agate_turb modifier can only be used with the agate pattern. See "Agate" for details.
The brick_size and mortar modifiers can only be used with the brick pattern. See "Brick" for details.
The control0 and control1 modifiers can only be used with the quilted pattern. See "Quilted" for details.
The interpolate modifier can only be used with the density_file pattern. See "Density_File" for details.
The general purpose pattern modifiers in the following sections can be used with pigment, normal, texture, or density patterns.
The most common pattern modifiers are the transformation modifiers translate, rotate, scale, transform, and matrix. For details on these commands see section "Transformations".
These modifiers may be placed inside pigment, normal, texture, and density statements to change the position, size and orientation of the patterns.
Transformations are performed in the order in which you specify them. However in general the order of transformations relative to other pattern modifiers such as turbulence, color_map and other maps is not important. For example scaling before or after turbulence makes no difference. The turbulence is done first, then the scaling regardless of which is specified first. However the order in which transformations are performed relative to warp statements is important. See "Warps" for details.
The frequency and phase modifiers act as a type of scale and translate modifiers for various blend maps. They only have effect when blend maps are used. Blend maps are color_map, pigment_map, normal_map, slope_map, density_map, and texture_map. This discussion uses a color map as an example but the same principles apply to the other blend map types.
The frequency keyword adjusts the number of times that a color map repeats over one cycle of a pattern. For example gradient covers color map values 0 to 1 over the range from x=0 to x=1. By adding frequency 2.0 the color map repeats twice over that same range. The same effect can be achieved using scale 0.5*x so the frequency keyword isn't that useful for patterns like gradient.
However the radial pattern wraps the color map around the +y-axis once. If you wanted two copies of the map (or 3 or 10 or 100) you'd have to build a bigger map. Adding frequency 2.0 causes the color map to be used twice per revolution. Try this:
pigment {
radial
color_map{[0.5 color Red][0.5 color White]}
frequency 6
}
The result is six sets of red and white radial stripes evenly spaced around the object.
The float after frequency can be any value. Values greater than 1.0 causes more than one copy of the map to be used. Values from 0.0 to 1.0 cause a fraction of the map to be used. Negative values reverses the map.
The phase value causes the map entries to be shifted so that the map starts and ends at a different place. In the example above if you render successive frames at phase 0 then phase 0.1, phase 0.2, etc. you could create an animation that rotates the stripes. The same effect can be easily achieved by rotating the radial pigment using rotate y*Angle but there are other uses where phase can be handy.
Sometimes you create a great looking gradient or wood color map but you want the grain slightly adjusted in or out. You could re-order the color map entries but that's a pain. A phase adjustment will shift everything but keep the same scale. Try animating a mandel pigment for a color palette rotation effect.
These values work by applying the following formula
New_Value = fmod ( Old_Value * Frequency + Phase, 1.0 ).
The frequency and phase modifiers have no effect on block patterns checker, brick, and hexagon nor do they effect image_map, bump_map or material_map. They also have no effect in normal statements when used with bumps, dents, quilted or wrinkles because these normal patterns cannot use normal_map or slope_map.
They can be used with normal patterns ripples and waves even though these two patterns cannot use normal_map or slope_map either. When used with ripples or waves, frequency adjusts the space between features and phase can be adjusted from 0.0 to 1.0 to cause the ripples or waves to move relative to their center for animating the features.
POV-Ray allows you to apply various wave forms to the pattern function before applying it to a blend map. Blend maps are color_map, pigment_map, normal_map, slope_map, density_map, and texture_map.
Most of the patterns which use a blend map, use the entries in the map in order from 0.0 to 1.0. The effect can most easily be seen when these patterns are used as normal patterns with no maps. Patterns such as gradient or onion generate a grove or slot that looks like a ramp that drops off sharply. This is called a ramp_wave wave type and it is the default wave type for most patterns. However the wood and marble patterns use the map from 0.0 to 1.0 and then reverses it and runs it from 1.0 to 0.0. The result is a wave form which slopes upwards to a peak, then slopes down again in a triangle_wave. In earlier versions of POV-Ray there was no way to change the wave types. You could simulate a triangle wave on a ramp wave pattern by duplicating the map entries in reverse, however there was no way to use a ramp wave on wood or marble.
Now any pattern that takes a map can have the default wave type overridden. For example:
pigment { wood color_map { MyMap } ramp_wave }
Also available are sine_wave, scallop_wave, cubic_wave and poly_wave types. These types are of most use in normal patterns as a type of built-in slope map. The sine_wave takes the zig-zag of a ramp wave and turns it into a gentle rolling wave with smooth transitions. The scallop_wave uses the absolute value of the sine wave which looks like corduroy when scaled small or like a stack of cylinders when scaled larger. The cubic_wave is a gentle cubic curve from 0.0 to 1.0 with zero slope at the start and end. The poly_wave is an exponential function. It is followed by an optional float value which specifies exponent. For example poly_wave 2 starts low and climbs rapidly at the end while poly_wave 0.5 climbs rapidly at first and levels off at the end. If no float value is specified, the default is 1.0 which produces a linear function identical to ramp_wave.
Although any of these wave types can be used for pigments, normals, textures, or density the effect of many of the wave types are not as noticeable on pigments, textures, or density as they are for normals.
Wave type modifiers have no effect on block patterns checker, brick, and hexagon nor do they effect image_map, bump_map or material_map. They also have no effect in normal statements when used with bumps, dents, quilted, ripples, waves, or wrinkles because these normal patterns cannot use normal_map or slope_map.
The keyword turbulence followed by a float or vector may be used to stir up any pigment, normal, texture, irid or density. A number of optional parameters may be used with turbulence to control how it is computed. The syntax is:
turbulence octaves Count | omega Amount | lambda Amount
Typical turbulence values range from the default 0.0, which is no turbulence, to 1.0 or more, which is very turbulent. If a vector is specified different amounts of turbulence are applied in the x-, y- and z-direction. For example
turbulence <1.0, 0.6, 0.1>
has much turbulence in the x-direction, a moderate amount in the y-direction and a small amount in the z-direction.
Turbulence uses a random noise function called DNoise. This is similar to the noise used in the bozo pattern except that instead of giving a single value it gives a direction. You can think of it as the direction that the wind is blowing at that spot. Points close together generate almost the same value but points far apart are randomly different.
In general the order of turbulence parameters relative to other pattern modifiers such as transformations, color maps and other maps is not important. For example scaling before or after turbulence makes no difference. The turbulence is done first, then the scaling regardless of which is specified first. See section "" for a way to work around this behavior.
In general, the order of turbulence parameters relative to each other and to other pattern modifiers such as transformations or color_map and other maps is not important. For example scaling before or after turbulence makes no difference. The turbulence is done first, then the scaling regardless of which is specified first. However the order in which transformations are performed relative to warp statements is important. You can also specify turbulence inside warp and in this way you can force turbulence to be applied after transformations. See "Warps" for details.
Turbulence uses DNoise to push a point around in several steps called octaves. We locate the point we want to evaluate, then push it around a bit using turbulence to get to a different point then look up the color or pattern of the new point.
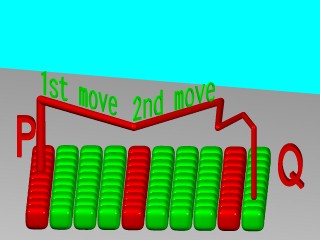
It says in effect "Don't give me the color at this spot... take a few random steps in different directions and give me that color". Each step is typically half as long as the one before. For example:

Turbulence random walk.
The magnitude of these steps is controlled by the turbulence value. There are three additional parameters which control how turbulence is computed. They are octaves, lambda and omega. Each is optional. Each is followed by a single float value. Each has no effect when there is no turbulence.
The octaves keyword may be followed by an integer value to control the number of steps of turbulence that are computed. Legal values range from 1 to 10. The default value of 6 is a fairly high value; you won't see much change by setting it to a higher value because the extra steps are too small. Float values are truncated to integer. Smaller numbers of octaves give a gentler, wavy turbulence and computes faster. Higher octaves create more jagged or fuzzy turbulence and takes longer to compute.
The lambda parameter controls how statistically different the random move of an octave is compared to its previous octave. The default value is 2.0 which is quite random. Values close to lambda 1.0 will straighten out the randomness of the path in the diagram above. The zig-zag steps in the calculation are in nearly the same direction. Higher values can look more swirly under some circumstances.
The omega value controls how large each successive octave step is compared to the previous value. Each successive octave of turbulence is multiplied by the omega value. The default omega 0.5 means that each octave is 1/2 the size of the previous one. Higher omega values mean that 2nd, 3rd, 4th and up octaves contribute more turbulence giving a sharper, crinkly look while smaller omegas give a fuzzy kind of turbulence that gets blurry in places.
The warp statement is a pattern modifier that is similar to turbulence. Turbulence works by taking the pattern evaluation point and pushing it about in a series of random steps. However warps push the point in very well-defined, non-random, geometric ways. The warp statement also overcomes some limitations of traditional turbulence and transformations by giving the user more control over the order in which turbulence, transformation and warp modifiers are applied to the pattern.
Currently there are three types of warps but the syntax was designed to allow future expansion. The first two, the repeat warp and the black_hole warp are new features for POV-Ray that modify the pattern in geometric ways. The other warp provides an alternative way to specify turbulence.
The syntax for using a warp statement is:
warp { }
repeat black_hole , Radius [BLACK_HOLE_ITEMS...] |
turbulence offset flip <Axis>
strength falloff Amount | inverse | type Type |
repeat turbulence <Amount>
octaves omega Amount | lambda Amount
You may have as many separate warp statements as you like in each pattern. The placement of warp statements relative to other modifiers such as color_map or turbulence is not important. However placement of warp statements relative to each other and to transformations is significant. Multiple warps and transformations are evaluated in the order in which you specify them. For example if you translate, then warp or warp, then translate, the results can be different.
A black_hole warp is so named because of its similarity to real black holes. Just like the real thing, you cannot actually see a black hole. The only way to detect its presence is by the effect it has on things that surround it.
Take, for example, a woodgrain. Using POV-Ray's normal turbulence and other texture modifier functions, you can get a nice, random appearance to the grain. But in its randomness it is regular - it is regularly random! Adding a black hole allows you to create a localized disturbance in a woodgrain in either one or multiple locations. The black hole can have the effect of either sucking the surrounding texture into itself (like the real thing) or pushing it away. In the latter case, applied to a woodgrain, it would look to the viewer as if there were a knothole in the wood. In this text we use a woodgrain regularly as an example, because it is ideally suitable to explaining black holes. However, black holes may in fact be used with any texture or pattern. The effect that the black hole has on the texture can be specified. By default, it sucks with the strength calculated exponentially (inverse-square). You can change this if you like.
Black holes may be used anywhere a warp is permitted. The syntax is:
warp {black_hole , Radius [BLACK_HOLE_ITEMS...] }
strength falloff Amount | inverse | type Type |
repeat turbulence <Amount>
The minimal requirement is the black_hole keyword followed by a vector <Location> followed by a comma and a float Radius. Black holes effect all points within the spherical region around the location and within the radius. This is optionally followed by any number of other keywords which control how the texture is warped.
The falloff keyword may be used with a float value to specify the power by which the effect of the black hole falls off. The default is two. The force of the black hole at any given point, before applying the strength modifier, is as follows.
First, convert the distance from the point to the center to a proportion (0 to 1) that the point is from the edge of the black hole. A point on the perimeter of the black hole will be 0.0; a point at the center will be 1.0; a point exactly halfway will be 0.5, and so forth. Mentally you can consider this to be a closeness factor. A closeness of 1.0 is as close as you can get to the center (i.e. at the center), a closeness of 0.0 is as far away as you can get from the center and still be inside the black hole and a closeness of 0.5 means the point is exactly halfway between the two.
Call this value c. Raise c to the power specified in falloff. By default Falloff is 2, so this is c2 or c squared. The resulting value is the force of the black hole at that exact location and is used, after applying the strength scaling factor as described below, to determine how much the point is perturbed in space. For example, if c is 0.5 the force is 0.52 or 0.25. If c is 0.25 the force is 0.125. But if c is exactly 1.0 the force is 1.0. Recall that as c gets smaller the point is farther from the center of the black hole. Using the default power of 2, you can see that as c reduces, the force reduces exponentially in an inverse-square relationship. Put in plain English, it means that the force is much stronger (by a power of two) towards the center than it is at the outside.
By increasing falloff, you can increase the magnitude of the falloff. A large value will mean points towards the perimeter will hardly be affected at all and points towards the center will be affected strongly. A value of 1.0 for falloff will mean that the effect is linear. A point that is exactly halfway to the center of the black hole will be affected by a force of exactly 0.5. A value of falloff of less than one but greater than zero means that as you get closer to the outside, the force increases rather than decreases. This can have some uses but there is a side effect. Recall that the effect of a black hole ceases outside its perimeter. This means that points just within the perimeter will be affected strongly and those just outside not at all. This would lead to a visible border, shaped as a sphere. A value for falloff of 0 would mean that the force would be 1.0 for all points within the black hole, since any number larger 0 raised to the power of 0 is 1.0.
The strength keyword may be specified with a float value to give you a bit more control over how much a point is perturbed by the black hole. Basically, the force of the black hole (as determined above) is multiplied by the value of strength, which defaults to 1.0. If you set strength to 0.5, for example, all points within the black hole will be moved by only half as much as they would have been. If you set it to 2.0 they will be moved twice as much.
There is a rider to the latter example, though - the movement is clipped to a maximum of the original distance from the center. That is to say, a point that is 0.75 units from the center may only be moved by a maximum of 0.75 units either towards the center or away from it, regardless of the value of strength. The result of this clipping is that you will have an exclusion area near the center of the black hole where all points whose final force value exceeded or equaled 1.0 were moved by a fixed amount.
If the inverted keyword is specified then points pushed away from the center instead of being pulled in.
The repeat keyword followed by a vector, allows you to simulate the effect of many black holes without having to explicitly declare them. Repeat is a vector that tells POV-Ray to use this black hole at multiple locations. Using repeat logically divides your scene up into cubes, the first being located at <0,0,0> and going to <Repeat>. Suppose your repeat vector was <1,5,2>. The first cube would be from <0,0,0> to < 1,5,2>. This cube repeats, so there would be one at < -1,-5,-2>, <1,5,2>, <2,10,4> and so forth in all directions, ad infinitum.
When you use repeat, the center of the black hole does not specify an absolute location in your scene but an offset into each block. It is only possible to use positive offsets. Negative values will produce undefined results.
Suppose your center was <0.5,1,0.25> and the repeat vector is <2,2,2>. This gives us a block at < 0,0,0> and <2,2,2>, etc. The centers of the black hole's for these blocks would be <0,0,0> + < 0.5,1.0,0.25>, i. e. <0.5,1.0,0.25>, and < 2,2,2> + <0.5,1.0,0.25>, i. e. < 2,5,3.0,2.25>.
Due to the way repeats are calculated internally, there is a restriction on the values you specify for the repeat vector. Basically, each black hole must be totally enclosed within each block (or cube), with no part crossing into a neighboring one. This means that, for each of the x, y and z dimensions, the offset of the center may not be less than the radius, and the repeat value for that dimension must be >=the center plus the radius since any other values would allow the black hole to cross a boundary. Put another way, for each of x, y and z
Radius <= Offset or Center <= Repeat - Radius.
If the repeat vector in any dimension is too small to fit this criteria, it will be increased and a warning message issued. If the center is less than the radius it will also be moved but no message will be issued.
Note that none of the above should be read to mean that you can't overlap black holes. You most certainly can and in fact this can produce some most useful effects. The restriction only applies to elements of the same black hole which is repeating. You can declare a second black hole that also repeats and its elements can quite happily overlap the first and causing the appropriate interactions. It is legal for the repeat value for any dimension to be 0, meaning that POV-Ray will not repeat the black hole in that direction.
The turbulence can only be used in a black hole with repeat. It allows an element of randomness to be inserted into the way the black holes repeat, to cause a more natural look. A good example would be an array of knotholes in wood - it would look rather artificial if each knothole were an exact distance from the previous.
The turbulence vector is a measurement that is added to each individual back hole in an array, after each axis of the vector is multiplied by a different random amount ranging from 0 to 1. The resulting actual position of the black hole's center for that particular repeat element is random (but consistent, so renders will be repeatable) and somewhere within the above co-ordinates. There is a rider on the use of turbulence, which basically is the same as that of the repeat vector. You can't specify a value which would cause a black hole to potentially cross outside of its particular block.
In summary: For each of x, y and z the offset of the center must be >=radius and the value of the repeat must be >= center + radius + turbulence. The exception being that repeat may be 0 for any dimension, which means do not repeat in that direction.
Some examples are given by
warp
{
black_hole <0, 0, 0>, 0.5
}
warp
{
black_hole <0.15, 0.125, 0>, 0.5
falloff 7
strength 1.0
repeat <1.25, 1.25, 0>
turbulence <0.25, 0.25, 0>
inverse
}
warp
{
black_hole <0, 0, 0>, 1.0
falloff 2
strength 2
inverse
}
The repeat warp causes a section of the pattern to be repeated over and over. It takes a slice out of the pattern and makes multiple copies of it side-by-side. The warp has many uses but was originally designed to make it easy to model wood veneer textures. Veneer is made by taking very thin slices from a log and placing them side-by-side on some other backing material. You see side-by-side nearly identical ring patterns but each will be a slice perhaps 1/32th of an inch deeper.
The syntax for a repeat warp is
warp { repeat <Directiont> [REPEAT_ITEMS...] }
offset flip <Axis>
The repeat vector specifies the direction in which the pattern repeats and the width of the repeated area. This vector must lie entirely along an axis. In other words, two of its three components must be 0. For example
pigment {
wood
warp {repeat 2*x}
}
which means that from x=0 to x=2 you get whatever the pattern usually is. But from x=2 to x=4 you get the same thing exactly shifted two units over in the x-direction. To evaluate it you simply take the x-coordinate modulo 2. Unfortunately you get exact duplicates which isn't very realistic. The optional offset vector tells how much to translate the pattern each time it repeats. For example
pigment {
wood
warp {repeat x*2 offset z*0.05}
}
means that we slice the first copy from x=0 to x=2 at z=0 but at x=2 to x=4 we offset to z=0.05. In the 4 to 6 interval we slice at z=0.10. At the n-th copy we slice at 0.05 n z. Thus each copy is slightly different. There are no restrictions on the offset vector.
Finally the flip vector causes the pattern to be flipped or mirrored every other copy of the pattern. The first copy of the pattern in the positive direction from the axis is not flipped. The next farther is, the next is not, etc. The flip vector is a three component x, y, z vector but each component is treated as a boolean value that tells if you should or should not flip along a given axis. For example
pigment {
wood
warp {repeat 2*x flip <1,1,0>}
}
means that every other copy of the pattern will be mirrored about the x- and y- axis but not the z-axis. A non-zero value means flip and zero means do not flip about that axis. The magnitude of the values in the flip vector doesn't matter.
The POV-Ray language contains an ambiguity and limitation on the way you specify turbulence and transformations such as translate, rotate, scale, matrix, and transform transforms. Usually the turbulence is done first. Then all translate, rotate, scale, matrix, and transform operations are always done after turbulence regardless of the order in which you specify them. For example this
pigment {
wood
scale .5
turbulence .2
}
works exactly the same as
pigment {
wood
turbulence .2
scale .5
}
The turbulence is always first. A better example of this limitation is with uneven turbulence and rotations.
pigment {
wood
turbulence 0.5*y
rotate z*60
}
// as compared to
pigment {
wood
rotate z*60
turbulence 0.5*y
}
The results will be the same either way even though you'd think it should look different.
We cannot change this basic behavior in POV-Ray now because lots of scenes would potentially render differently if suddenly the order transformation vs turbulence suddenly mattered when in the past, it didn't.
However, by specifying our turbulence inside warp statement you tell POV-Ray that the order in which turbulence, transformations and other warps are applied is significant. Here's an example of a turbulence warp.
warp { turbulence <0,1,1> octaves 3 lambda 1.5 omega 0.3 }
The significance is that this
pigment {
wood
translate <1,2,3> rotate x*45 scale 2
warp { turbulence <0,1,1> octaves 3 lambda 1.5 omega 0.3 }
}
produces different results than this...
pigment {
wood
warp { turbulence <0,1,1> octaves 3 lambda 1.5 omega 0.3 }
translate <1,2,3> rotate x*45 scale 2
}
You may specify turbulence without using a warp statement. However you cannot control the order in which they are evaluated unless you put them in a warp.
The evaluation rules are as follows:
1) First any turbulence not inside a warp statement is applied regardless of the order in which it appears relative to warps or transformations.
2) Next each warp statement, translate, rotate, scale or matrix one-by-one, is applied in the order the user specifies. If you want turbulence done in a specific order, you simply specify it inside a warp in the proper place.
A bitmap modifier is a modifier used inside an image_map, bump_map or material_map to specify how the 2-D bitmap is to be applied to the 3-D surface. Several bitmap modifiers apply to specific kinds of maps and they are covered in the appropriate sections. The bitmap modifiers discussed in the following sections are applicable to all three types of bitmaps.
Normally there are an infinite number of repeating image maps, bump maps or material maps created over every unit square of the x-y-plane like tiles. By adding the once keyword after a file name you can eliminate all other copies of the map except the one at (0,0) to (1,1). In image maps, areas outside this unit square are treated as fully transparent. In bump maps, areas outside this unit square are left flat with no normal modification. In material maps, areas outside this unit square are textured with the first texture of the texture list.
For example:
image_map {
gif "mypic.gif"
once
}
The default projection of the image onto the x-y-plane is called a planar map type. This option may be changed by adding the map_type keyword followed by an integer number specifying the way to wrap the image around the object.
A map_type 0 gives the default planar mapping already described.
A map_type 1 gives a spherical mapping. It assumes that the object is a sphere of any size sitting at the origin. The y-axis is the north/south pole of the spherical mapping. The top and bottom edges of the image just touch the pole regardless of any scaling. The left edge of the image begins at the positive x-axis and wraps the image around the sphere from west to east in a -y-rotation. The image covers the sphere exactly once. The once keyword has no meaning for this mapping type.
With map_type 2 you get a cylindrical mapping. It assumes that a cylinder of any diameter lies along the y-axis. The image wraps around the cylinder just like the spherical map but the image remains one unit tall from y=0 to y=1. This band of color is repeated at all heights unless the once keyword is applied.
Finally map_type 5 is a torus or donut shaped mapping. It assumes that a torus of major radius one sits at the origin in the x-z-plane. The image is wrapped around similar to spherical or cylindrical maps. However the top and bottom edges of the map wrap over and under the torus where they meet each other on the inner rim.
Types 3 and 4 are still under development.
Note that the map_type option may also be applied to bump_map and material_map statements.
For example:
sphere{<0,0,0>,1
pigment{
image_map {
gif "world.gif"
map_type 1
}
}
}
Adding the interpolate keyword can smooth the jagged look of a bitmap. When POV-Ray asks an image map color or a bump amount for a bump map, it often asks for a point that is not directly on top of one pixel but sort of between several differently colored pixels. Interpolations returns an in-between value so that the steps between the pixels in the map will look smoother.
Although interpolate is legal in material maps, the color index is interpolated before the texture is chosen. It does not interpolate the final color as you might hope it would. In general, interpolation of material maps serves no useful purpose but this may be fixed in future versions.
There are currently two types of interpolation: interpolate 2 gives bilinear interpolation while interpolate 4 gives normalized distance. For example:
image_map {
gif "mypic.gif"
interpolate 2
}
Default is no interpolation. Normalized distance is the slightly faster of the two, bilinear does a better job of picking the between color. Normally bilinear is used.
If your map looks jaggy, try using interpolation instead of going to a higher resolution image. The results can be very good.